Mathematics

A Little Connection between Geometry and Number Theory

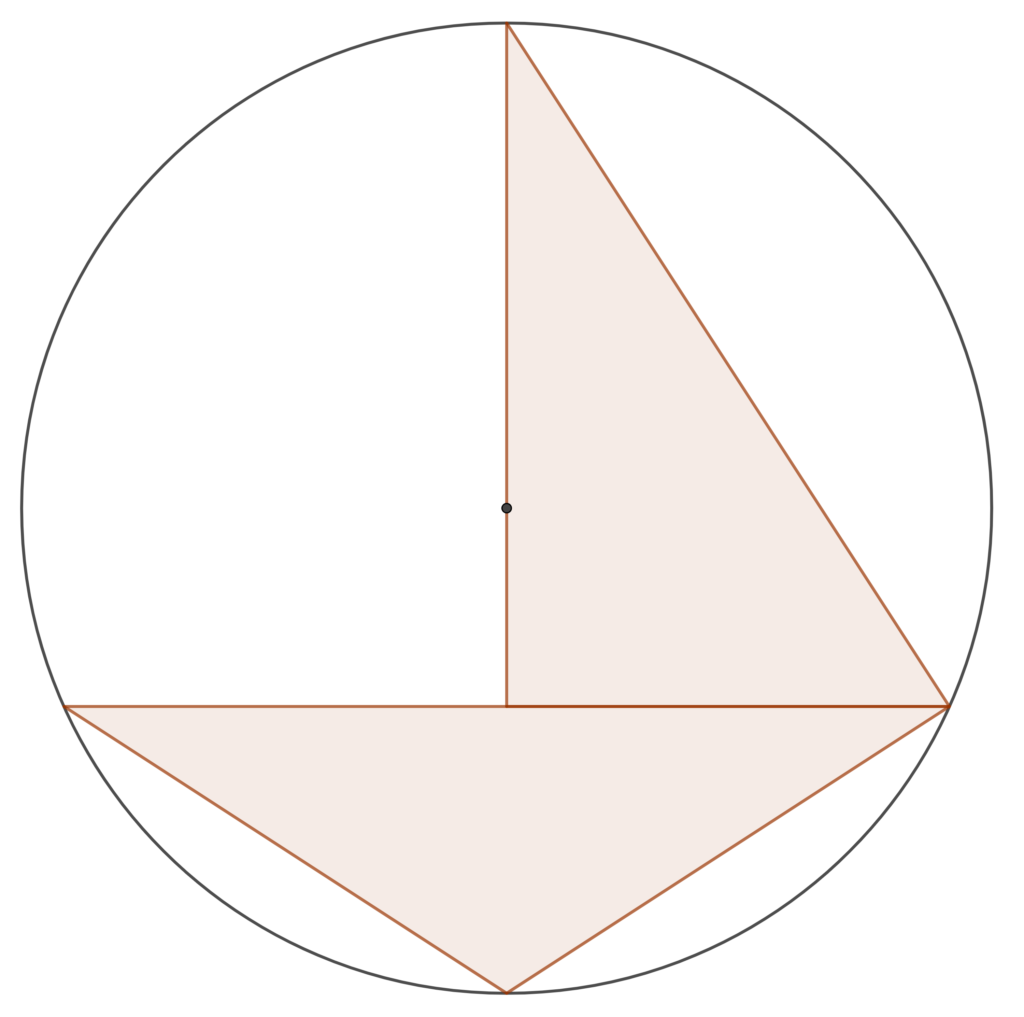
In this geometry problem an isosceles triangle and a right-angled triangle are inscribed in a circle. When you have solved the problem the answer may surprise you, as it has a connection to number theory. The short side of the right-angled triangle has length one and it is coincident with half of the base of the isosceles triangle which has length two. The constraint that defines the problem is that the perimeters of the two triangles are equal. What are the lengths of the other sides and what is the radius of the circle? The diagram shows that the right-angled corner is at the midpoint of the base of the isosceles triangle and that the centre of the circle is a point on the perpendicular side of the right angled triangle.

January 23, 2023 by Geogebra Geometry Mathematics 0

Two Mutually Tangent Circles in a Rectangle

In this geometry problem two circles of different sizes and two line segments of equal length fit precisely in a rectangle. Given that the smaller circle has radius 1, what is the radius r of the larger circle? The circles are mutually tangent at point C as depicted. The unit circle on the left is tangent to two sides of the rectangle, and the larger circle of radius r is tangent to three of the sides. The line segment OD connects the centres of the circles. The rectangle has been adjusted so that the distance from the point of tangency C to the nearest corner P is equal to the length of OD. Can you develop an equation in its simplest form for the radius r? Additionally, express the width and height of the rectangle in terms of r.

August 1, 2022 by Geometry Mathematics 0

Two Discs in an Envelope

Two circles are inscribed in a rectangle as shown. One diagonal passes through the point of tangency where the two circles touch, and the other diagonal is tangent to the smaller circle.

You may define the radius of the large circle as 1 and the radius of the small circle as r. Can you find an equation for r, and numeric values for r and for the lengths of the sides of the rectangle?

I created this construction using Geogebra, iteratively adjusting it until everything fitted. The aspect ratio of the rectangle turns out to be about 1.72, a fact that can be used to check your own result. The dimensions of the rectangle are not an initial condition, but a consequence of the radii of the two circles. However, as an alternative exercise try drawing this construction yourself, beginning with a 100 by 172 rectangle.

I am delighted to report that this problem was solved independently by Ted Courant and by Keith Raskin after I posted it on the Math, Math Education, Math Culture group on LinkedIn. They also observed that the rectangle is more accurately drawn with side lengths 1003 and 1725.

May 22, 2022 by Geogebra Geometry Mathematics 1